Being not too happy with the speed of the shared hosting, decided to move the blog to an Amazon Elastic Compute Cloud (Amazon EC2) instance. Given this is a baby step, picked up a micro instance running an Ubuntu server and installed Apache web server, MySQL, PHP . After doing a bit of tweaking with this new…
In this post, we will explore a probable way of reducing PAPR (peak to average power ratio) in OFDM by changing the phase of some of the subcarriers. This is in response to the comment to post on Peak to Average power ratio for OFDM, where Mr. Elibom suggested to reduce the PAPR by cyclically…
Mr. Raghuram contacted me and informed about Happy Schools Blog. He writes about Graduate School Admission in U.S., Job opportunities for students, University Selection based on his personal experience. He recently published few articles which might of interest to some of our readers. Here are the URL for few articles:
Coding is a technique where redundancy is added to original bit sequence to increase the reliability of the communication. In this article, lets discuss a simple binary convolutional coding scheme at the transmitter and the associated Viterbi (maximum likelihood) decoding scheme at the receiver. Update: For some reason, the blog is unable to display the…
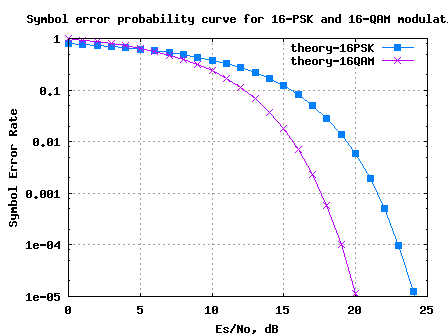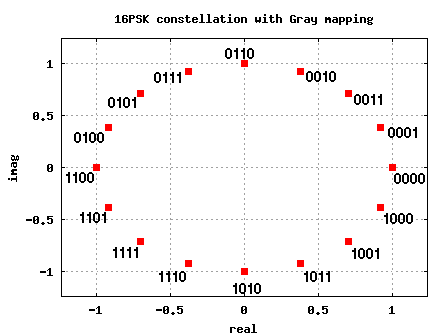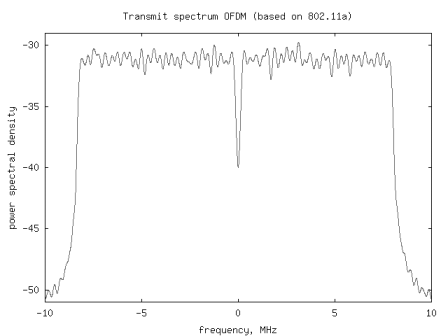
In this post, let us try to understand Gray codes and their usage in digital communication. Quoting from Wiki entry on Gray code [Gray-Wiki], The reflected binary code, also known as Gray code after Frank Gray, is a binary numeral system where two successive values differ in only one digit. In a digital communication system,…
In this post, let us evaluate the impact of frequency offset resulting in Inter Carrier Interference (ICI) while receiving an OFDM modulated symbol. We will first discuss the OFDM transmission and reception, the effect of frequency offset and later we will define the loss of orthogonality and resulting signal to noise ratio (SNR) loss due…
In previous posts, we had discussed equalization of a 2×2 MIMO channel with Zero Forcing (ZF) equalization and later, Zero Forcing equalization with successive interference cancellation (ZF-SIC). In this post, we will explore a variant of ZF-SIC called Zero Forcing Successive Interference Cancellation with optimal ordering. We will assume that the channel is a flat…
Given that we have discussed symbol error rate probability for a 4-PAM modulation, let us know focus on finding the symbol error probability for a QPSK (4-QAM) modulation scheme. Background Consider that the alphabets used for a QPSK (4-QAM) is (Refer example 5-35 in [DIG-COMM-BARRY-LEE-MESSERSCHMITT]). Download free e-Book discussing theoretical and simulated error rates for…
Long back in time we discussed the BER (bit error rate) for BPSK modulation in a simple AWGN channel (time stamps states August 2007). Almost an year back! It high time we discuss the BER for BPSK in a Rayleigh multipath channel. In a brief discussion on Rayleigh channel, wherein we stated that a circularly…
Given that we have went over the symbol error probability for 4-PAM and symbol error rate for 4-QAM , let us extend the understanding to find the symbol error probability for 16-QAM (16 Quadrature Amplitude Modulation). Consider a typical 16-QAM modulation scheme where the alphabets (Refer example 5-37 in [DIG-COMM-BARRY-LEE-MESSERSCHMITT]). are used.
Let us try to understand simulation of a typical Orthogonal Frequency Division Multiplexing (OFDM) transmission defined per IEEE 802.11a specification. Orthogonal pulses In a previous post (here ), we have understood that the minimum frequency separation for two sinusoidals with arbitrary phases to be orthogonal is , where is the symbol period. In Orthogonal Frequency…
Let us derive the theoretical 16QAM bit error rate (BER) with Gray coded constellation mapping in additive white Gaussian noise conditions. Further, the Matlab/Octave simulation script can be used to confirm that the simulation is in good agreement with theory.
In a previous post (here), we looked at using CORDIC (Co-ordinate Rotation by DIgital Computer) for understanding how a complex number can be rotated by an angle without using actual multipliers. Let us know try to understand how we can use CORDIC for finding the phase and magnitude of a complex number. Basics The CORDIC…